1. Introduction to Calculus
What is Calculus?
Calculus is the branch of mathematics that deals with change. Unlike algebra, which is static, calculus allows us to understand and describe changes. It’s a powerful tool used in various fields such as physics, engineering, economics, and even biology.
Why Learn Calculus?
Learning calculus opens up a new world of mathematical understanding. It provides the foundation for more advanced studies in mathematics and science. Whether you’re looking to pursue a career in a STEM field or just want to challenge yourself, calculus is a great place to start.
2. The Building Blocks of Calculus
Functions and Their Importance
At its core, calculus deals with functions. A function is a relationship between two variables where each input is related to exactly one output. Understanding functions is crucial as they form the basis of most calculus problems.
Different Types of Functions
There are various types of functions you’ll encounter in calculus. These include linear functions, polynomial functions, exponential functions, and trigonometric functions. Each type behaves differently and has unique properties.
3. Limits: The Foundation of Calculus
Understanding Limits
Limits are the cornerstone of calculus. They help us understand the behavior of functions as they approach a particular point. Limits can be tricky, but they are essential for defining derivatives and integrals.
Calculating Limits
To calculate a limit, you approach the value from both sides and see what the function tends to. Sometimes, you can find limits algebraically, while other times you might need to use a graph or numerical methods.
4. Derivatives: Measuring Change
What is a Derivative?
A derivative represents the rate of change of a function with respect to a variable. In simpler terms, it tells you how fast something is changing at any given point. Derivatives are fundamental in understanding motion, growth, and other dynamic processes.
Basic Rules of Differentiation
There are several rules to make finding derivatives easier. These include the power rule, product rule, quotient rule, and chain rule. Mastering these rules is essential for solving more complex problems.
5. Applications of Derivatives
Motion and Velocity
One of the most common applications of derivatives is in physics, where they are used to describe motion. The derivative of the position function with respect to time gives you the velocity, which tells you how fast an object is moving.
Optimization Problems
Derivatives are also used in optimization problems. These problems involve finding the maximum or minimum values of a function, which is essential in fields like economics and engineering.
6. Integrals: Accumulating Change
What is an Integral?
An integral is the opposite of a derivative. While derivatives measure the rate of change, integrals measure the total accumulation of change. Integrals are used to calculate areas under curves, among other things.
Basic Integration Techniques
There are several techniques for finding integrals, including substitution and integration by parts. Learning these techniques is crucial for solving a wide range of problems.
7. The Fundamental Theorem of Calculus
Connecting Derivatives and Integrals
The Fundamental Theorem of Calculus links the concepts of differentiation and integration. It states that differentiation and integration are inverse processes. This theorem is a central idea in calculus and has profound implications.
Practical Implications
Understanding this theorem allows you to solve complex problems more efficiently. It shows that if you know the antiderivative of a function, you can easily compute the integral.
8. Differential Equations
What are Differential Equations?
Differential equations involve equations that relate a function to its derivatives. They are used to model a wide range of real-world phenomena, from population growth to the motion of planets.
Solving Simple Differential Equations
Solving differential equations can be challenging, but many techniques can simplify the process. For beginners, focusing on first-order differential equations is a good starting point.
9. Series and Sequences
Understanding Sequences
A sequence is an ordered list of numbers that often follow a specific pattern. Sequences are the building blocks of series, which are sums of sequences. Understanding sequences is important for studying series and their convergence.
Convergence and Divergence
A series can either converge (approach a finite value) or diverge (grow without bound). Knowing whether a series converges or diverges is crucial in many areas of mathematics and applied science.
10. Multivariable Calculus
Extending Calculus to Multiple Variables
Multivariable calculus extends the concepts of single-variable calculus to functions of several variables. This includes studying partial derivatives and multiple integrals.
Applications in the Real World
Multivariable calculus is used in many fields, such as physics for describing electromagnetic fields, in economics for optimizing functions with several variables, and in engineering for analyzing systems with multiple inputs.
11. Tips for Studying Calculus
Practice Regularly
The key to mastering calculus is practice. Work on problems regularly to build your understanding and improve your problem-solving skills. Don’t just stick to examples in textbooks—challenge yourself with new and different problems.
Seek Help When Needed
Don’t be afraid to seek help when you’re stuck. Use resources like online tutorials, study groups, and tutoring services. Sometimes, a different perspective can make a complex topic much clearer.
12. Real-World Applications of Calculus
Engineering and Physics
Calculus is indispensable in engineering and physics. It’s used to design and analyze systems, from the simple mechanics of a lever to the complex dynamics of a spacecraft.
Economics and Biology
In economics, calculus helps in modeling and predicting economic trends. In biology, it’s used to model population dynamics and the spread of diseases.
13. Technology and Calculus
Calculus in Computer Science
Calculus plays a crucial role in computer science, particularly in areas like machine learning and algorithms. Understanding calculus can give you a significant advantage in this rapidly growing field.
Software Tools for Calculus
There are numerous software tools available that can help you with calculus. These include graphing calculators, computer algebra systems, and educational apps that provide interactive problem-solving experiences.
14. Historical Perspectives
The Origins of Calculus
Calculus has a rich history that dates back to ancient times. However, it was formalized in the 17th century by mathematicians Isaac Newton and Gottfried Wilhelm Leibniz. Understanding the historical context can give you a deeper appreciation of the subject.
Key Mathematicians
Many mathematicians have contributed to the development of calculus. Learning about their lives and work can inspire you and provide valuable insights into the subject.
15. Advanced Topics in Calculus
Vector Calculus
Vector calculus deals with vector fields and is essential in physics and engineering. It extends the concepts of calculus to more complex systems and provides powerful tools for analyzing them.
Fourier Analysis
Fourier analysis is a method of expressing functions as sums of sinusoidal functions. It’s widely used in signal processing, image analysis, and other fields that require analyzing periodic data.
16. Calculus in Everyday Life
Calculus in Medicine
In medicine, calculus is used in various ways, such as modeling the growth of tumors or the spread of diseases. It’s also used in medical imaging techniques like MRI and CT scans.
Environmental Science
Environmental scientists use calculus to model and predict changes in the environment. This includes studying climate change, pollution levels, and the dynamics of ecosystems.
17. Learning Resources
Textbooks and Online Courses
There are many excellent textbooks and online courses available for learning calculus. Some popular ones include “Calculus” by James Stewart and online courses from platforms like Khan Academy and Coursera.
Interactive Learning
Interactive learning tools, such as simulations and educational software, can make studying calculus more engaging and effective. These tools allow you to visualize concepts and practice problems in a hands-on way.
18. Common Mistakes and How to Avoid Them
Misunderstanding Limits
One common mistake is misunderstanding the concept of limits. It’s essential to grasp this fundamental idea as it underpins much of calculus. Practice different types of limit problems to build a strong foundation.
Skipping Steps
Another mistake is skipping steps in problem-solving. Calculus problems can be complex, and skipping steps often leads to errors. Write out each step clearly to ensure you understand the process.
19. Preparing for Calculus Exams
Study Strategies
Effective study strategies include reviewing notes regularly, practicing problems, and understanding key concepts rather than memorizing formulas. Creating a study schedule can help manage your time efficiently.
Practice Exams
Taking practice exams can help you prepare for the format and types of questions you’ll encounter. It also helps you identify areas where you need further review and practice.
20. Calculus and Beyond
Transitioning to Advanced Mathematics
Calculus is a gateway to more advanced areas of mathematics, such as differential equations, linear algebra, and complex analysis. Mastering calculus prepares you for these more challenging subjects.
Career Opportunities
A strong understanding of calculus opens up many career opportunities in fields like engineering, data science, economics, and more. It’s a valuable skill that can set you apart in the job market.
21. Conclusion: Embracing the Journey
The Joy of Learning Calculus
Learning calculus can be challenging, but it’s also incredibly rewarding. The skills and knowledge you gain will serve you well in many areas of your life and career. Calculus is just the beginning. Keep exploring and learning new mathematical concepts. The journey of learning never ends, and each new topic builds on the foundation you’ve established with calculus.

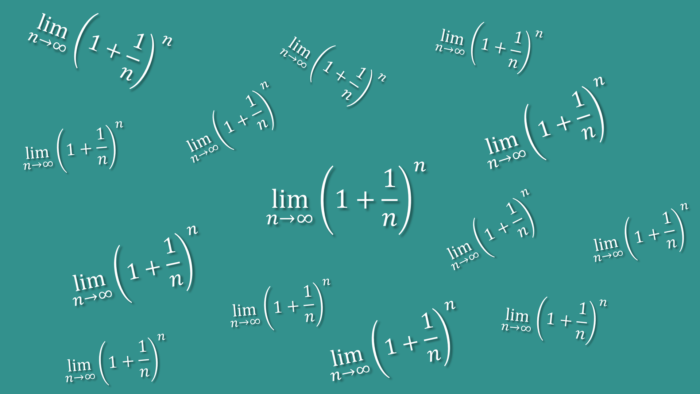
Excellent article. I am going through some of these issues as well..
My web page; nordvpn coupons inspiresensation
nordvpn coupon code 350fairfax
Greetings from Ohio! I’m bored to tears at work so I decided to browse your site on my iphone during lunch break.
I love the info you provide here and can’t wait to take a look when I get home.
I’m shocked at how fast your blog loaded on my phone ..
I’m not even using WIFI, just 3G .. Anyways, excellent site!